Physical geodesy: Difference between revisions
add nav. bar |
en>FrB.TG m Undid revision 586067835 by Advancedxu (talk) |
||
| Line 1: | Line 1: | ||
{{Use dmy dates|date=July 2013}} | |||
[[File:Error Function.svg|thumb|right|400px|Plot of the error function]] | |||
In [[mathematics]], the '''error function''' (also called the '''Gauss error function''') is a [[special function]] (non-[[Elementary function|elementary]]) of [[Sigmoid function|sigmoid]] shape which occurs in [[probability]], [[statistics]] and [[partial differential equation]]s. It is defined as:<ref>Andrews, Larry C.; [http://books.google.co.uk/books?id=2CAqsF-RebgC&pg=PA110#v=onepage&q&f=false ''Special functions of mathematics for engineers'']</ref><ref name="Greene">Greene, William H.; ''Econometric Analysis'' (fifth edition), Prentice-Hall, 1993, p. 926, fn. 11</ref> | |||
:<math>\operatorname{erf}(x) = \frac{2}{\sqrt{\pi}}\int_{0}^x e^{-t^2}\,\mathrm dt.</math> | |||
The '''complementary error function''', denoted ''erfc'', is defined as | |||
:<math>\begin{align} | |||
\operatorname{erfc}(x) & = 1-\operatorname{erf}(x) \\ | |||
& = \frac{2}{\sqrt{\pi}} \int_x^{\infty} e^{-t^2}\,\mathrm dt = e^{-x^2} \operatorname{erfcx}(x), | |||
\end{align} </math> | |||
which also defines ''erfcx'', the '''scaled complementary error function'''<ref name=Cody93>W. J. Cody, "Algorithm 715: SPECFUN—A portable FORTRAN package of special function routines and test drivers," ''ACM Trans. Math. Soft.'' '''19''', pp. 22–32 (1993).</ref> (which can be used instead of erfc to avoid [[arithmetic underflow]]<ref name=Cody93/><ref name=Zaghloul07>M. R. Zaghloul, "On the calculation of the Voigt line profile: a single proper integral with a damped sine integrand," ''Monthly Notices of the Royal Astronomical Society'' '''375'', pp. 1043–1048 (2007).</ref>). | |||
The '''imaginary error function''', denoted ''erfi'', is defined as | |||
:<math>\operatorname{erfi}(z) = -i\operatorname{erf}(iz) = \frac{2}{\sqrt{\pi}} e^{x^2} D(x)</math>, | |||
where ''D''(''x'') is the [[Dawson function]] (which can be used instead of erfi to avoid [[arithmetic overflow]]<ref name=Cody93/>). | |||
When the error function is evaluated for arbitrary [[complex number|complex]] arguments ''z'', the resulting '''complex error function''' is usually discussed in scaled form as the [[Faddeeva function]]: | |||
:<math>w(z) = e^{-z^2}\operatorname{erfc}(-iz) = \operatorname{erfcx}(-iz).</math> | |||
==The name "error function"== | |||
The error function is used in measurement theory (using probability and statistics), and although its use in other branches of mathematics has nothing to do with the characterization of measurement errors, the name has stuck. | |||
The error function is related to the cumulative distribution <math>\Phi</math>, the integral of the [[standard normal distribution]], by<ref name="Greene" /> | |||
:<math>\Phi (x) = \frac{1}{2}+ \frac{1}{2} \operatorname{erf} \left(x/ \sqrt{2}\right).</math> | |||
The error function, evaluated at <math>\frac{x}{\sigma \sqrt{2}}</math> for positive ''x'' values, gives the probability that a measurement, under the influence of normally distributed errors with [[standard deviation]] <math>\sigma</math>, has a distance less than x from the mean value.<ref>Van Zeghbroeck, Bart; ''Principles of Semiconductor Devices'', University of Colorado, 2011. [http://ecee.colorado.edu/~bart/book/gaussian.htm]</ref> This function is used in statistics to predict behavior of any sample with respect to the population mean. This usage is similar to the [[Q-function]], which in fact can be written in terms of the error function. | |||
==Properties== | |||
{{multiple image | |||
| header = Plots in the complex plane | |||
| direction = vertical | |||
| width = 250 | |||
| image1 = ComplexEx2.jpg | |||
| caption1 = Integrand exp(−''z''<sup>2</sup>) | |||
| image2 = ComplexErf.jpg | |||
| caption2 = erf(''z'') | |||
}} | |||
The property <math>\operatorname{erf} (-z) = -\operatorname{erf} (z)</math> means that the error function is an [[even and odd functions|odd function]]. | |||
For any [[complex number]] ''z'': | |||
:<math>\operatorname{erf} (\overline{z}) = \overline{\operatorname{erf}(z)} </math> | |||
where <math>\overline{z}</math> is the [[complex conjugate]] of ''z''. | |||
The integrand ''ƒ'' = exp(−''z''<sup>2</sup>) and ''ƒ'' = erf(''z'') are shown in the complex ''z''-plane in figures 2 and 3. Level of Im(''ƒ'') = 0 is shown with a thick green line. Negative integer values of Im(''ƒ'') are shown with thick red lines. Positive integer values of Im(''f'') are shown with thick blue lines. Intermediate levels of Im(''ƒ'') = constant are shown with thin green lines. Intermediate levels of Re(''ƒ'') = constant are shown with thin red lines for negative values and with thin blue lines for positive values. | |||
At the real axis, erf(''z'') approaches unity at ''z'' → +∞ and −1 at ''z'' → −∞. At the imaginary axis, it tends to ±i∞. | |||
<!-- ; the relation <math>{\rm erf}(-z)=-{\rm erf}(z)</math> holds.!--> | |||
===Taylor series=== | |||
The error function is an [[entire function]]; it has no singularities (except that at infinity) and its [[Taylor expansion]] always converges. | |||
The defining integral cannot be evaluated in [[Closed-form expression|closed form]] in terms of [[Elementary function (differential algebra)|elementary functions]], but by expanding the [[integrand]] ''e''<sup>−''z''<sup>2</sup></sup> into its Taylor series and integrating term by term, one obtains the error function's Taylor series as: | |||
:<math>\operatorname{erf}(z)= \frac{2}{\sqrt{\pi}}\sum_{n=0}^\infin\frac{(-1)^n z^{2n+1}}{n! (2n+1)} =\frac{2}{\sqrt{\pi}} \left(z-\frac{z^3}{3}+\frac{z^5}{10}-\frac{z^7}{42}+\frac{z^9}{216}-\ \cdots\right)</math> | |||
which holds for every [[complex number]] ''z''. The denominator terms are sequence [[oeis:A007680|A007680]] in the [[OEIS]]. | |||
For iterative calculation of the above series, the following alternative formulation may be useful: | |||
:<math>\operatorname{erf}(z)= \frac{2}{\sqrt{\pi}}\sum_{n=0}^\infin\left(z \prod_{k=1}^n {\frac{-(2k-1) z^2}{k (2k+1)}}\right) = \frac{2}{\sqrt{\pi}} \sum_{n=0}^\infin \frac{z}{2n+1} \prod_{k=1}^n \frac{-z^2}{k}</math> | |||
because <math>\frac{-(2k-1) z^2}{k (2k+1)}</math> expresses the multiplier to turn the ''k''<sup>th</sup> term into the (''k'' + 1)<sup>th</sup> term (considering ''z'' as the first term). | |||
The error function at +∞ is exactly 1 (see [[Gaussian integral]]). | |||
The derivative of the error function follows immediately from its definition: | |||
:<math>\frac{\rm d}{{\rm d}z}\,\mathrm{erf}(z)=\frac{2}{\sqrt{\pi}}\,e^{-z^2}.</math> | |||
An [[antiderivative]] of the error function is | |||
:<math>z\,\operatorname{erf}(z) + \frac{e^{-z^2}}{\sqrt{\pi}}.</math> | |||
===Inverse functions=== | |||
The '''inverse error function''' can be defined in terms of the [[Maclaurin series]] | |||
:<math>\operatorname{erf}^{-1}(z)=\sum_{k=0}^\infin\frac{c_k}{2k+1}\left (\frac{\sqrt{\pi}}{2}z\right )^{2k+1}, \,\!</math> | |||
where ''c''<sub>0</sub> = 1 and | |||
:<math>c_k=\sum_{m=0}^{k-1}\frac{c_m c_{k-1-m}}{(m+1)(2m+1)} = \left\{1,1,\frac{7}{6},\frac{127}{90},\frac{4369}{2520},\ldots\right\}.</math> | |||
So we have the series expansion (note that common factors have been canceled from numerators and denominators): | |||
:<math>\operatorname{erf}^{-1}(z)=\tfrac{1}{2}\sqrt{\pi}\left (z+\frac{\pi}{12}z^3+\frac{7\pi^2}{480}z^5+\frac{127\pi^3}{40320}z^7+\frac{4369\pi^4}{5806080}z^9+\frac{34807\pi^5}{182476800}z^{11}+\cdots\right ).\ </math> | |||
(After cancellation the numerator/denominator fractions are entries A092676/A132467 in the [[OEIS]]; without cancellation the numerator terms are given in entry A002067.) Note that the error function's value at ±∞ is equal to ±1. | |||
The '''inverse complementary error function''' is defined as | |||
:<math>\operatorname{erfc}^{-1}(1-z) = \operatorname{erf}^{-1}(z).</math> | |||
===Asymptotic expansion=== | |||
A useful [[asymptotic expansion]] of the complementary error function (and therefore also of the error function) for large real ''x'' is | |||
:<math>\mathrm{erfc}(x) = \frac{e^{-x^2}}{x\sqrt{\pi}}\left [1+\sum_{n=1}^\infty (-1)^n \frac{1\cdot3\cdot5\cdots(2n-1)}{(2x^2)^n}\right ]=\frac{e^{-x^2}}{x\sqrt{\pi}}\sum_{n=0}^\infty (-1)^n \frac{(2n-1)!!}{(2x^2)^n},\,</math> | |||
where (2''n'' – 1)!! is the [[double factorial]]: the product of all odd numbers up to (2''n'' – 1). This series diverges for every finite ''x'', and its meaning as asymptotic expansion is that, for any <math>N\in\N</math> one has | |||
:<math>\mathrm{erfc}(x) = \frac{e^{-x^2}}{x\sqrt{\pi}}\sum_{n=0}^{N-1} (-1)^n \frac{(2n-1)!!}{(2x^2)^n}+ R_N(x) \,</math> | |||
where the remainder, in [[Landau notation]], is | |||
:<math>R_N(x)=O(x^{-2N+1} e^{-x^2})</math> as <math>x\to\infty</math>. | |||
Indeed, the exact value of the remainder is | |||
:<math>R_N(x):= \frac{(-1)^N}{\sqrt{\pi}}2^{-2N+1}\frac{(2N)!}{N!}\int_x^\infty t^{-2N}e^{-t^2}\,\mathrm dt, | |||
</math> | |||
which follows easily by induction, writing <math>e^{-t^2}=-(2t)^{-1}(e^{-t^2})'</math> and integrating by parts. | |||
For large enough values of x, only the first few terms of this asymptotic expansion are needed to obtain a good approximation of erfc(''x'') (while for not too large values of ''x'' note that the above Taylor expansion at 0 provides a very fast convergence). | |||
===Continued fraction expansion=== | |||
A continued fraction expansion of the complementary error function is:<ref>{{cite book | |||
| last1 = Cuyt | |||
| first1 = Annie A. M. | |||
| last2 = Petersen | |||
| first2 = Vigdis B. | |||
| last3 = Verdonk | |||
| first3 = Brigitte | |||
| last4 = Waadeland | |||
| first4 = Haakon | |||
| last5 = Jones | |||
| first5 = William B. | |||
| title = Handbook of Continued Fractions for Special Functions | |||
| publisher = [[Springer-Verlag]] | |||
| year = 2008 | |||
| isbn = 978-1-4020-6948-2 | |||
}}</ref> | |||
: <math>\mathrm{erfc}(z) = \frac{z}{\sqrt{\pi}}e^{-z^2} | |||
\cfrac{a_1}{z^2+ | |||
\cfrac{a_2}{1+ | |||
\cfrac{a_3}{z^2+ | |||
\cfrac{a_4}{1+\dotsb}}}} | |||
\qquad a_1 = 1,\quad a_m = \frac{m-1}{2},\quad m \geq 2. | |||
</math> | |||
==Approximation with elementary functions== | |||
[[Abramowitz and Stegun]] give several approximations of varying accuracy (equations 7.1.25–28). This allows one to choose the fastest approximation suitable for a given application. In order of increasing accuracy, they are: | |||
: <math>\operatorname{erf}(x)\approx 1-\frac{1}{(1+a_1x+a_2x^2+a_3x^3+a_4x^4)^4}</math> (maximum error: 5·10<sup>−4</sup>) | |||
where ''a''<sub>1</sub> = 0.278393, ''a''<sub>2</sub> = 0.230389, ''a''<sub>3</sub> = 0.000972, ''a''<sub>4</sub> = 0.078108 | |||
: <math>\operatorname{erf}(x)\approx 1-(a_1t+a_2t^2+a_3t^3)e^{-x^2},\quad t=\frac{1}{1+px}</math> (maximum error: 2.5·10<sup>−5</sup>) | |||
where ''p'' = 0.47047, ''a''<sub>1</sub> = 0.3480242, ''a''<sub>2</sub> = −0.0958798, ''a''<sub>3</sub> = 0.7478556 | |||
: <math>\operatorname{erf}(x)\approx 1-\frac{1}{(1+a_1x+a_2x^2+\cdots+a_6x^6)^{16}}</math> (maximum error: 3·10<sup>−7</sup>) | |||
where ''a''<sub>1</sub> = 0.0705230784, ''a''<sub>2</sub> = 0.0422820123, ''a''<sub>3</sub> = 0.0092705272, ''a''<sub>4</sub> = 0.0001520143, ''a''<sub>5</sub> = 0.0002765672, ''a''<sub>6</sub> = 0.0000430638 | |||
: <math>\operatorname{erf}(x)\approx 1-(a_1t+a_2t^2+\cdots+a_5t^5)e^{-x^2},\quad t=\frac{1}{1+px}</math> (maximum error: 1.5·10<sup>−7</sup>) | |||
where ''p'' = 0.3275911, ''a''<sub>1</sub> = 0.254829592, ''a''<sub>2</sub> = −0.284496736, ''a''<sub>3</sub> = 1.421413741, ''a''<sub>4</sub> = −1.453152027, ''a''<sub>5</sub> = 1.061405429 | |||
All of these approximations are valid for ''x'' ≥ 0. To use these approximations for negative ''x'', use the fact that erf(x) is an odd function, so erf(''x'') = −erf(−''x''). | |||
Another approximation is given by | |||
: <math>\operatorname{erf}(x)\approx \sgn(x) \sqrt{1-\exp\left(-x^2\frac{4/\pi+ax^2}{1+ax^2}\right)}</math> | |||
where | |||
: <math>a = \frac{8(\pi-3)}{3\pi(4-\pi)} \approx 0.140012.</math> | |||
<!-- | |||
The range of approximation and the precision are not reported; the fitting may take place in vicinity of the real axis. --> | |||
This is designed to be very accurate in a neighborhood of 0 and a neighborhood of infinity, and the error is less than 0.00035 for all ''x''. Using the alternate value ''a'' ≈ 0.147 reduces the maximum error to about 0.00012.<ref>{{Cite web |last=Winitzki |first=Sergei |date=6 February 2008 |title=A handy approximation for the error function and its inverse |url=http://sites.google.com/site/winitzki/sergei-winitzkis-files/erf-approx.pdf |format=PDF |accessdate=2011-10-03 }}</ref><!-- Note: a=0.14784 gives a maximum error of ~.000104, better than a = 0.147 --> | |||
This approximation can also be inverted to calculate the inverse error function: | |||
:<math>\operatorname{erf}^{-1}(x)\approx \sgn(x) \sqrt{\sqrt{\left(\frac{2}{\pi a}+\frac{\ln(1-x^2)}{2}\right)^2 - \frac{\ln(1-x^2)}{a}} | |||
-\left(\frac{2}{\pi a}+\frac{\ln(1-x^2)}{2}\right)}.</math> | |||
A pure exponential approximation for the complementary error function is given by {{harvtxt|Chiani|Dardari|Simon|2003}} | |||
: <math>\operatorname{erfc}(x)\approx \frac{1}{6}e^{-x^2}+\frac{1}{2}e^{-\frac{4}{3} x^2} \qquad x>0 \,.</math> | |||
== Numerical approximation == | |||
Over the complete range of values, there is an approximation with a maximal error of <math>1.2\cdot10^{-7}</math>, as follows:<ref>Numerical Recipes in Fortran 77: The Art of Scientific Computing (ISBN 0-521-43064-X), 1992, page 214, Cambridge University Press.</ref> | |||
:<math>\operatorname{erf}(x)=\begin{cases} | |||
1-\tau & \mathrm{for\;}x\ge 0\\ | |||
\tau-1 & \mathrm{for\;}x < 0 | |||
\end{cases}</math> | |||
with | |||
:<math>\begin{array}{rcl} | |||
\tau & = & t\cdot\exp\left(-x^{2}-1.26551223+1.00002368\cdot t+0.37409196\cdot t^{2}+0.09678418\cdot t^{3}\right.\\ | |||
& & \qquad-0.18628806\cdot t^{4}+0.27886807\cdot t^{5}-1.13520398\cdot t^{6}+1.48851587\cdot t^7\\ | |||
& & \qquad\left.-0.82215223\cdot t^{8}+0.17087277\cdot t^{9}\right) | |||
\end{array}</math> | |||
and | |||
:<math>t=\frac{1}{1+0.5\,|x|}</math> | |||
==Applications== | |||
When the results of a series of measurements are described by a [[normal distribution]] with [[standard deviation]] <math>\scriptstyle\sigma</math> and [[expected value]] 0, then <math> \scriptstyle\operatorname{erf}\,\left(\,\frac{a}{\sigma \sqrt{2}}\,\right)</math> is the probability that the error of a single measurement lies between −''a'' and +''a'', for positive ''a''. This is useful, for example, in determining the [[bit error rate]] of a digital communication system. | |||
The error and complementary error functions occur, for example, in solutions of the [[heat equation]] when [[boundary condition]]s are given by the [[Heaviside step function]]. | |||
==Related functions== | |||
The error function is essentially identical to the standard [[normal cumulative distribution function]], denoted Φ, also named norm(x) by software languages, as they differ only by scaling and translation. Indeed, | |||
: <math>\Phi(x) =\frac{1}{\sqrt{2\pi}}\int_{-\infty}^x e^\tfrac{-t^2}{2}\,\mathrm dt = \frac{1}{2}\left[1+\operatorname{erf}\left(\frac{x}{\sqrt{2}}\right)\right]=\frac{1}{2}\,\operatorname{erfc}\left(-\frac{x}{\sqrt{2}}\right)</math> | |||
or rearranged for erf and erfc: | |||
:<math>\begin{align} | |||
\mathrm{erf}(x) &= 2 \Phi \left ( x \sqrt{2} \right ) - 1 \\ | |||
\mathrm{erfc}(x) &= 2 \Phi \left ( - x \sqrt{2} \right )=2\left(1-\Phi \left ( x \sqrt{2} \right)\right). | |||
\end{align}</math> | |||
Consequently, the error function is also closely related to the [[Q-function]], which is the tail probability of the standard normal distribution. The Q-function can be expressed in terms of the error function as | |||
:<math> | |||
Q(x) =\frac{1}{2} - \frac{1}{2} \operatorname{erf} \left( \frac{x}{\sqrt{2}} \right)=\frac{1}{2}\operatorname{erfc}\left(\frac{x}{\sqrt{2}}\right). | |||
</math> | |||
The [[inverse function|inverse]] of <math>\scriptstyle\Phi\,</math> is known as the [[Quantile function|normal quantile function]], or [[probit]] function and may be expressed in terms of the inverse error function as | |||
:<math> | |||
\operatorname{probit}(p) = \Phi^{-1}(p) = \sqrt{2}\,\operatorname{erf}^{-1}(2p-1) = -\sqrt{2}\,\operatorname{erfc}^{-1}(2p). | |||
</math> | |||
The standard normal cdf is used more often in probability and statistics, and the error function is used more often in other branches of mathematics. | |||
The error function is a special case of the [[Mittag-Leffler function]], and can also be expressed as a [[confluent hypergeometric function]] (Kummer's function): | |||
:<math>\mathrm{erf}(x)= | |||
\frac{2x}{\sqrt{\pi}}\,_1F_1\left(\tfrac12,\tfrac32,-x^2\right).</math> | |||
It has a simple expression in terms of the [[Fresnel integral]].{{Elucidate|date=May 2012}} | |||
In terms of the [[Incomplete_Gamma_function#Regularized_Gamma_functions|Regularized Gamma function P]] and the [[incomplete gamma function]], | |||
:<math>\operatorname{erf}(x)=\operatorname{sgn}(x) P\left(\tfrac12, x^2\right)={\operatorname{sgn}(x) \over \sqrt{\pi}}\gamma\left(\tfrac12, x^2\right).</math> | |||
<math>\scriptstyle\operatorname{sgn}(x) \ </math> is the [[sign function]]. | |||
===Generalized error functions=== | |||
[[File:Error Function Generalised.svg|right|thumb|400px|Graph of generalised error functions ''E''<sub>n</sub>(''x''):<br /> | |||
grey curve: ''E''<sub>1</sub>(''x'') = (1 − e<sup> −''x''</sup>)/<math>\scriptstyle\sqrt{\pi}</math><br /> | |||
red curve: ''E''<sub>2</sub>(''x'') = erf(''x'')<br /> | |||
green curve: ''E''<sub>3</sub>(''x'')<br /> | |||
blue curve: ''E''<sub>4</sub>(''x'')<br /> | |||
gold curve: ''E''<sub>5</sub>(''x'').]] | |||
Some authors discuss the more general functions:{{citation needed|date=August 2011}} | |||
:<math>E_n(x) = \frac{n!}{\sqrt{\pi}} \int_0^x e^{-t^n}\,\mathrm dt | |||
=\frac{n!}{\sqrt{\pi}}\sum_{p=0}^\infin(-1)^p\frac{x^{np+1}}{(np+1)p!}\,.</math> | |||
Notable cases are: | |||
*''E''<sub>0</sub>(''x'') is a straight line through the origin: <math>\scriptstyle E_0(x)=\frac{x}{e \sqrt{\pi}}</math> | |||
*''E''<sub>2</sub>(''x'') is the error function, erf(''x''). | |||
After division by ''n''!, all the ''E<sub>n</sub>'' for odd ''n'' look similar (but not identical) to each other. Similarly, the ''E<sub>n</sub>'' for even ''n'' look similar (but not identical) to each other after a simple division by ''n''!. All generalised error functions for ''n'' > 0 look similar on the positive ''x'' side of the graph. | |||
These generalised functions can equivalently be expressed for ''x'' > 0 using the [[Gamma function]] and [[incomplete Gamma function]]: | |||
:<math>E_n(x) = \frac{\Gamma(n)\left(\Gamma\left(\frac{1}{n}\right)-\Gamma\left(\frac{1}{n},x^n\right)\right)}{\sqrt\pi}, | |||
\quad \quad | |||
x>0.\ </math> | |||
Therefore, we can define the error function in terms of the incomplete Gamma function: | |||
:<math>\operatorname{erf}(x) = 1 - \frac{\Gamma\left(\frac{1}{2},x^2\right)}{\sqrt\pi}.\ </math> | |||
===Iterated integrals of the complementary error function=== | |||
The iterated integrals of the complementary error function are defined by | |||
:<math> | |||
\mathrm i^n \operatorname{erfc}\, (z) = \int_z^\infty \mathrm i^{n-1} \operatorname{erfc}\, (\zeta)\;\mathrm d \zeta.\, | |||
</math> | |||
They have the power series | |||
:<math> | |||
\mathrm i^n \operatorname{erfc}\, (z) | |||
= | |||
\sum_{j=0}^\infty \frac{(-z)^j}{2^{n-j}j! \Gamma \left( 1 + \frac{n-j}{2}\right)}\,, | |||
</math> | |||
from which follow the symmetry properties | |||
:<math> | |||
\mathrm i^{2m} \operatorname{erfc} (-z) | |||
= - \mathrm i^{2m} \operatorname{erfc}\, (z) | |||
+ \sum_{q=0}^m \frac{z^{2q}}{2^{2(m-q)-1}(2q)! (m-q)!} | |||
</math> | |||
and | |||
:<math> | |||
\mathrm i^{2m+1} \operatorname{erfc} (-z) | |||
= \mathrm i^{2m+1} \operatorname{erfc}\, (z) | |||
+ \sum_{q=0}^m \frac{z^{2q+1}}{2^{2(m-q)-1}(2q+1)! (m-q)!}\,. | |||
</math> | |||
==Implementations== | |||
*[[C (programming language)|C]]: C99 provides the functions <tt>double erf(double x)</tt> and <tt>double erfc(double x)</tt> in the header [[math.h]]. The pairs of functions {<tt>erff()</tt>,<tt>erfcf()</tt>} and {<tt>erfl()</tt>,<tt>erfcl()</tt>} take and return values of type <tt>float</tt> and <tt>long double</tt> respectively. For <tt>complex double</tt> arguments, the function names <tt>cerf</tt> and <tt>cerfc</tt> are "reserved for future use"; the missing implementation is provided by the open-source project [http://apps.jcns.fz-juelich.de/libcerf libcerf], which is based on the [http://ab-initio.mit.edu/Faddeeva Faddeeva package]. | |||
*[[C++]]: C++11 provides <tt>erf()</tt> and <tt>erfc()</tt> in the header <tt>cmath</tt>. Both functions are overloaded to accept arguments of type <tt>float</tt>, <tt>double</tt>, and <tt>long double</tt>. For <tt>complex<double></tt>, the [http://ab-initio.mit.edu/Faddeeva Faddeeva package] provides a C++ <tt>complex<double></tt> implementation. | |||
*[[Fortran]]: The Fortran 2008 standard provides the <tt>ERF</tt>, <tt>ERFC</tt> and <tt>ERFC_SCALED</tt> functions to calculate the error function and its complement for real arguments. [[Fortran 77]] implementations are available in [[SLATEC]]. | |||
*[[Google Search|Google search]]: Google's search also acts as a calculator and will evaluate "erf(...)" and "erfc(...)" for real arguments. | |||
*[[Haskell (programming language)|Haskell]]: An erf package<ref>http://hackage.haskell.org/package/erf</ref> exists that provides a typeclass for the error function and implementations for the native (real) floating point types. | |||
*[[IDL (programming language)| IDL]]: provides both erf and erfc for real and complex arguments. | |||
*[[Java (programming language)|Java]]: Apache commons-math<ref>http://commons.apache.org/math</ref> provides implementations of erf and erfc for real arguments. | |||
*[[Maple (software)|Maple]]: Maple implements both erf and erfc for real and complex arguments. | |||
*[[MathCAD]] provides both erf(x) and erfc(x) for real arguments. | |||
*[[Mathematica]]: erf is implemented as Erf and Erfc in Mathematica for real and complex arguments, which are also available in [[Wolfram Alpha]]. | |||
*[[Matlab]] provides both erf and erfc for real arguments, also via W. J. Cody's algorithm.<ref name=cody69/> | |||
*[[Maxima (software)|Maxima]] provides both erf and erfc for real and complex arguments. | |||
*[[Perl]]: erf (for real arguments, using Cody's algorithm<ref name=cody69/>) is implemented in the Perl module Math::SpecFun | |||
*[[Python (programming language)|Python]]: Included since version 2.7 as <code>math.erf()</code> for real arguments. For previous versions or for complex arguments, [[SciPy]] includes implementations of erf, erfc, erfi, and related functions for complex arguments in <code>scipy.special</code>.<ref>[http://docs.scipy.org/doc/scipy/reference/special.html#error-function-and-fresnel-integrals Error Function and Fresnel Integrals], SciPy v0.13.0 Reference Guide.</ref> A complex-argument erf is also in the [[arbitrary-precision arithmetic]] mpmath library as <code>mpmath.erf()</code> | |||
*[[R (programming language)|R]]: "The so-called 'error function'"<ref>{{citation |title=R: The Normal Distribution |url=http://stat.ethz.ch/R-manual/R-patched/library/stats/html/Normal.html |author=R Development Core Team |date=25 February 2011 }}</ref> is not provided directly, but is detailed as an example of the [[normal cumulative distribution function]] (<code>?pnorm</code>), which is based on W. J. Cody's rational Chebyshev approximation algorithm.<ref name=cody69>{{cite journal |last=Cody |first=William J. |year=1969 |title=Rational Chebyshev Approximations for the Error Function |journal=Math. Comp. |volume=23 |issue=107 |pages=631–637 |doi=10.1090/S0025-5718-1969-0247736-4 }}</ref> | |||
*[[Ruby (programming language)|Ruby]]: Provides <code>Math.erf()</code> and <code>Math.erfc()</code> for real arguments. | |||
==See also== | |||
=== Related functions === | |||
* [[Gaussian integral]], over the whole real line | |||
* [[Gaussian function]], derivative | |||
* [[Dawson function]], renormalized imaginary error function | |||
====In probability==== | |||
* [[Normal distribution]] | |||
* [[Normal cumulative distribution function]], a scaled and shifted form of error function | |||
* [[Probit]], the inverse or [[quantile function]] of the normal CDF | |||
* [[Q-function]], the tail probability of the normal distribution | |||
==References== | |||
{{Reflist}} | |||
* {{AS ref |7|297}} | |||
*{{Citation |last1=Press |first1=William H. |last2=Teukolsky |first2=Saul A. |last3=Vetterling |first3=William T. |last4=Flannery |first4=Brian P. |year=2007 |title=Numerical Recipes: The Art of Scientific Computing |edition=3rd |publisher=Cambridge University Press |publication-place=New York |isbn=978-0-521-88068-8 |chapter=Section 6.2. Incomplete Gamma Function and Error Function |chapter-url=http://apps.nrbook.com/empanel/index.html#pg=259 }} | |||
*{{dlmf|id=7|title=Error Functions, Dawson’s and Fresnel Integrals|first=Nico M. |last=Temme }} | |||
*{{Citation | |||
|last1=Chiani | first1=M. | |||
|last2=Dardari | first2=D. | |||
|last3=Simon | first3=M. K. | |||
|year=2003 | |||
|title = New Exponential Bounds and Approximations for the Computation of Error Probability in Fading Channels | |||
| journal=[[IEEE Transactions on Wireless Communications]] | |||
| volume=4 | issue=2 | pages=840–845 | |||
| doi=10.1109/TWC.2003.814350 | |||
}}. | |||
==External links== | |||
* [http://mathworld.wolfram.com/Erf.html MathWorld – Erf] | |||
* [http://www.miniwebtool.com/error-function-calculator/ Error-function numerical table and calculator] | |||
{{DEFAULTSORT:Error Function}} | |||
[[Category:Special hypergeometric functions]] | |||
[[Category:Gaussian function]] | |||
[[Category:Functions related to probability distributions]] | |||
[[Category:Analytic functions]] | |||
Latest revision as of 19:01, 14 December 2013
30 year-old Entertainer or Range Artist Wesley from Drumheller, really loves vehicle, property developers properties for sale in singapore singapore and horse racing. Finds inspiration by traveling to Works of Antoni Gaudí.
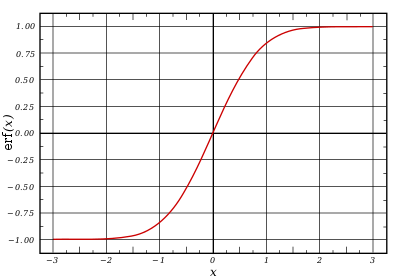
In mathematics, the error function (also called the Gauss error function) is a special function (non-elementary) of sigmoid shape which occurs in probability, statistics and partial differential equations. It is defined as:[1][2]
The complementary error function, denoted erfc, is defined as
which also defines erfcx, the scaled complementary error function[3] (which can be used instead of erfc to avoid arithmetic underflow[3][4]).
The imaginary error function, denoted erfi, is defined as
where D(x) is the Dawson function (which can be used instead of erfi to avoid arithmetic overflow[3]).
When the error function is evaluated for arbitrary complex arguments z, the resulting complex error function is usually discussed in scaled form as the Faddeeva function:
The name "error function"
The error function is used in measurement theory (using probability and statistics), and although its use in other branches of mathematics has nothing to do with the characterization of measurement errors, the name has stuck.
The error function is related to the cumulative distribution , the integral of the standard normal distribution, by[2]
The error function, evaluated at for positive x values, gives the probability that a measurement, under the influence of normally distributed errors with standard deviation , has a distance less than x from the mean value.[5] This function is used in statistics to predict behavior of any sample with respect to the population mean. This usage is similar to the Q-function, which in fact can be written in terms of the error function.
Properties
People are nonetheless rushing to buy properties in Singapore. A new residential condominium mission in Bedok had a long queue three days before its official launch and bought more than 350 units on the very first day. Nonetheless, there is a ‘development' that had emerged in 2011 that the majority have possibly overlooked.
In August, more than a hundred individuals across 16 states reportedly had been sickened with salmonella after consuming mangos In September, the Meals and Drug Administration detained mango imports from a Mexican packing house after the fruits had been linked to the sickenings Save Cash On Your Online Purchasing Make saving money when purchasing on-line a breeze with Bodge's favourite app, Invisible Hands. This helpful program does the price-checking analysis for you, and will warn you when a unique site has a better value - or let you realize that you have found the most effective deal. Watch Posted by Edison Foo September 29, 2013 HEARTH SALE!!! From only $9xx,000! We hope this listing will help launch your search for new properties in Singapore. It's unlawful to purchase ice cream after 6 pm, in Newark.
for a borrower with one or more excellent residential property loans3; and No SSD will be payable by the seller if the property is bought more than three years after it was bought. Non-extension of the Jan 2009 Funds assistance measures for the property market when the measures expire. Permitting up to 2 years of property tax deferral for land accepted for improvement. Lively secondary marketplace for easy exit of property investments. Curiosity on mortgage fee is tax deductible when the property is rented out. We've progressive property tax rates for residential properties- the higher the value of the residential property, the upper the tax charge. Why do I've to pay property tax on my house when I'm an onwer-occupier and not deriving rental earnings? Palacio Cluster Landed
After obtaining a Sale License (topic to authorities circumstances meant to guard individuals shopping for property in Singapore), he might www.rmmonline.com proceed to sell units in his growth. Funding in the property market of Singapore is likely one of the few investment choices the place utilizing the bank's cash could not be any easier. The power of expats, to make a down payment, leverage the capital and consequently improve total return on investment, is excessive in Singapore. PRs who own a HDB flat must sell their flat inside six months of buying a non-public residential property in Singapore. EVERLASTING residents (PRs) now face unprecedented limits on their skill to purchase property in Singapore. New Condo in 2013 March Foreigners are eligible for Singapore greenback mortgage loan. housing grant
Skilled property brokers in Singapore will assist the client and defend their interests throughout the acquisition, and will help safe the provide at the best possible price. The agents may even be sure that all paperwork are in order and that the vendor is the rightful proprietor of the property. Most of the property brokers share their databases with each other. Tuesday's measures come after the Monetary Authority of Singapore in June tightened rules on property loans, and closed loopholes that allowed some householders to circumvent loan restrictions and avoid paying levies when buying extra property. Contact us for a FREE housing loan analysis and to study concerning the newest mortgage to worth on Singapore housing loans. J Gateway Condominium (Bought Out) Oct, 2013
6. Completion of automotive park, roads and drains serving the housing project 5% 7. Notice of Vacant Possession (Upon obtaining Temporary Occupation Permit) The estimates given by SingaporeHousingLoan.SG's calculator are not quotations and are usually not binding in any way on SingaporeHousingLoan.sg or the consumer. The method of calculation does not exactly mirror that of which is being used by the individual monetary establishment and the data provided should be used as a information only. SingaporeHousingLoan.SG's calculator does not pre-qualify you for any housing loan from any monetary institution. Undercover Report Finds Unlawful Rat Meat Sold In London Market Unpasteurized Tempeh Linked To Salmonella Outbreak That Sickens 60 Hottest EC Launch in Sengkang!
BuyRentSingaporeProperty.com is dedicated in providing the newest residential launches, Developer's sales, new launches, resale, HIGH tasks and rental of properties in Singapore. Then there are new launch property condos in the mid-tier range. These are sometimes in the vicinity of the city fringe, in areas corresponding to Newton and Novena Tanjong Rhu. These new launch projects are popular due to their proximity to town heart, the place the enterprise and entertainment centers and all the amenities they provide, but they're usually cheaper than the City core region tasks. Why submit cheques to register for new property launches in Singapore? Riversails Apartment @ Higher Serangoon LakeVille @ Jurong Lakeside Condo by MCL Ferra Condo @ Leonie Hill
The property means that the error function is an odd function.
For any complex number z:
where is the complex conjugate of z.
The integrand ƒ = exp(−z2) and ƒ = erf(z) are shown in the complex z-plane in figures 2 and 3. Level of Im(ƒ) = 0 is shown with a thick green line. Negative integer values of Im(ƒ) are shown with thick red lines. Positive integer values of Im(f) are shown with thick blue lines. Intermediate levels of Im(ƒ) = constant are shown with thin green lines. Intermediate levels of Re(ƒ) = constant are shown with thin red lines for negative values and with thin blue lines for positive values.
At the real axis, erf(z) approaches unity at z → +∞ and −1 at z → −∞. At the imaginary axis, it tends to ±i∞.
Taylor series
The error function is an entire function; it has no singularities (except that at infinity) and its Taylor expansion always converges.
The defining integral cannot be evaluated in closed form in terms of elementary functions, but by expanding the integrand e−z2 into its Taylor series and integrating term by term, one obtains the error function's Taylor series as:
which holds for every complex number z. The denominator terms are sequence A007680 in the OEIS.
For iterative calculation of the above series, the following alternative formulation may be useful:
because expresses the multiplier to turn the kth term into the (k + 1)th term (considering z as the first term).
The error function at +∞ is exactly 1 (see Gaussian integral).
The derivative of the error function follows immediately from its definition:
An antiderivative of the error function is
Inverse functions
The inverse error function can be defined in terms of the Maclaurin series
where c0 = 1 and
So we have the series expansion (note that common factors have been canceled from numerators and denominators):
(After cancellation the numerator/denominator fractions are entries A092676/A132467 in the OEIS; without cancellation the numerator terms are given in entry A002067.) Note that the error function's value at ±∞ is equal to ±1.
The inverse complementary error function is defined as
Asymptotic expansion
A useful asymptotic expansion of the complementary error function (and therefore also of the error function) for large real x is
where (2n – 1)!! is the double factorial: the product of all odd numbers up to (2n – 1). This series diverges for every finite x, and its meaning as asymptotic expansion is that, for any one has
where the remainder, in Landau notation, is
Indeed, the exact value of the remainder is
which follows easily by induction, writing and integrating by parts.
For large enough values of x, only the first few terms of this asymptotic expansion are needed to obtain a good approximation of erfc(x) (while for not too large values of x note that the above Taylor expansion at 0 provides a very fast convergence).
Continued fraction expansion
A continued fraction expansion of the complementary error function is:[6]
Approximation with elementary functions
Abramowitz and Stegun give several approximations of varying accuracy (equations 7.1.25–28). This allows one to choose the fastest approximation suitable for a given application. In order of increasing accuracy, they are:
where a1 = 0.278393, a2 = 0.230389, a3 = 0.000972, a4 = 0.078108
where p = 0.47047, a1 = 0.3480242, a2 = −0.0958798, a3 = 0.7478556
where a1 = 0.0705230784, a2 = 0.0422820123, a3 = 0.0092705272, a4 = 0.0001520143, a5 = 0.0002765672, a6 = 0.0000430638
where p = 0.3275911, a1 = 0.254829592, a2 = −0.284496736, a3 = 1.421413741, a4 = −1.453152027, a5 = 1.061405429
All of these approximations are valid for x ≥ 0. To use these approximations for negative x, use the fact that erf(x) is an odd function, so erf(x) = −erf(−x).
Another approximation is given by
where
This is designed to be very accurate in a neighborhood of 0 and a neighborhood of infinity, and the error is less than 0.00035 for all x. Using the alternate value a ≈ 0.147 reduces the maximum error to about 0.00012.[7]
This approximation can also be inverted to calculate the inverse error function:
A pure exponential approximation for the complementary error function is given by Template:Harvtxt
Numerical approximation
Over the complete range of values, there is an approximation with a maximal error of , as follows:[8]
with
and
Applications
When the results of a series of measurements are described by a normal distribution with standard deviation and expected value 0, then is the probability that the error of a single measurement lies between −a and +a, for positive a. This is useful, for example, in determining the bit error rate of a digital communication system.
The error and complementary error functions occur, for example, in solutions of the heat equation when boundary conditions are given by the Heaviside step function.
Related functions
The error function is essentially identical to the standard normal cumulative distribution function, denoted Φ, also named norm(x) by software languages, as they differ only by scaling and translation. Indeed,
or rearranged for erf and erfc:
Consequently, the error function is also closely related to the Q-function, which is the tail probability of the standard normal distribution. The Q-function can be expressed in terms of the error function as
The inverse of is known as the normal quantile function, or probit function and may be expressed in terms of the inverse error function as
The standard normal cdf is used more often in probability and statistics, and the error function is used more often in other branches of mathematics.
The error function is a special case of the Mittag-Leffler function, and can also be expressed as a confluent hypergeometric function (Kummer's function):
It has a simple expression in terms of the Fresnel integral.Template:Elucidate
In terms of the Regularized Gamma function P and the incomplete gamma function,
is the sign function.
Generalized error functions

grey curve: E1(x) = (1 − e −x)/
red curve: E2(x) = erf(x)
green curve: E3(x)
blue curve: E4(x)
gold curve: E5(x).
Some authors discuss the more general functions:Potter or Ceramic Artist Truman Bedell from Rexton, has interests which include ceramics, best property developers in singapore developers in singapore and scrabble. Was especially enthused after visiting Alejandro de Humboldt National Park.
Notable cases are:
After division by n!, all the En for odd n look similar (but not identical) to each other. Similarly, the En for even n look similar (but not identical) to each other after a simple division by n!. All generalised error functions for n > 0 look similar on the positive x side of the graph.
These generalised functions can equivalently be expressed for x > 0 using the Gamma function and incomplete Gamma function:
Therefore, we can define the error function in terms of the incomplete Gamma function:
Iterated integrals of the complementary error function
The iterated integrals of the complementary error function are defined by
They have the power series
from which follow the symmetry properties
and
Implementations
- C: C99 provides the functions double erf(double x) and double erfc(double x) in the header math.h. The pairs of functions {erff(),erfcf()} and {erfl(),erfcl()} take and return values of type float and long double respectively. For complex double arguments, the function names cerf and cerfc are "reserved for future use"; the missing implementation is provided by the open-source project libcerf, which is based on the Faddeeva package.
- C++: C++11 provides erf() and erfc() in the header cmath. Both functions are overloaded to accept arguments of type float, double, and long double. For complex<double>, the Faddeeva package provides a C++ complex<double> implementation.
- Fortran: The Fortran 2008 standard provides the ERF, ERFC and ERFC_SCALED functions to calculate the error function and its complement for real arguments. Fortran 77 implementations are available in SLATEC.
- Google search: Google's search also acts as a calculator and will evaluate "erf(...)" and "erfc(...)" for real arguments.
- Haskell: An erf package[9] exists that provides a typeclass for the error function and implementations for the native (real) floating point types.
- IDL: provides both erf and erfc for real and complex arguments.
- Maple: Maple implements both erf and erfc for real and complex arguments.
- MathCAD provides both erf(x) and erfc(x) for real arguments.
- Mathematica: erf is implemented as Erf and Erfc in Mathematica for real and complex arguments, which are also available in Wolfram Alpha.
- Maxima provides both erf and erfc for real and complex arguments.
- Perl: erf (for real arguments, using Cody's algorithm[11]) is implemented in the Perl module Math::SpecFun
- Python: Included since version 2.7 as
math.erf()for real arguments. For previous versions or for complex arguments, SciPy includes implementations of erf, erfc, erfi, and related functions for complex arguments inscipy.special.[12] A complex-argument erf is also in the arbitrary-precision arithmetic mpmath library asmpmath.erf()
- R: "The so-called 'error function'"[13] is not provided directly, but is detailed as an example of the normal cumulative distribution function (
?pnorm), which is based on W. J. Cody's rational Chebyshev approximation algorithm.[11]
- Ruby: Provides
Math.erf()andMath.erfc()for real arguments.
See also
Related functions
- Gaussian integral, over the whole real line
- Gaussian function, derivative
- Dawson function, renormalized imaginary error function
In probability
- Normal distribution
- Normal cumulative distribution function, a scaled and shifted form of error function
- Probit, the inverse or quantile function of the normal CDF
- Q-function, the tail probability of the normal distribution
References
43 year old Petroleum Engineer Harry from Deep River, usually spends time with hobbies and interests like renting movies, property developers in singapore new condominium and vehicle racing. Constantly enjoys going to destinations like Camino Real de Tierra Adentro.
- Template:AS ref
- Many property agents need to declare for the PIC grant in Singapore. However, not all of them know find out how to do the correct process for getting this PIC scheme from the IRAS. There are a number of steps that you need to do before your software can be approved.
Naturally, you will have to pay a safety deposit and that is usually one month rent for annually of the settlement. That is the place your good religion deposit will likely be taken into account and will kind part or all of your security deposit. Anticipate to have a proportionate amount deducted out of your deposit if something is discovered to be damaged if you move out. It's best to you'll want to test the inventory drawn up by the owner, which can detail all objects in the property and their condition. If you happen to fail to notice any harm not already mentioned within the inventory before transferring in, you danger having to pay for it yourself.
In case you are in search of an actual estate or Singapore property agent on-line, you simply should belief your intuition. It's because you do not know which agent is nice and which agent will not be. Carry out research on several brokers by looking out the internet. As soon as if you end up positive that a selected agent is dependable and reliable, you can choose to utilize his partnerise in finding you a home in Singapore. Most of the time, a property agent is taken into account to be good if he or she locations the contact data on his website. This may mean that the agent does not mind you calling them and asking them any questions relating to new properties in singapore in Singapore. After chatting with them you too can see them in their office after taking an appointment.
Have handed an trade examination i.e Widespread Examination for House Brokers (CEHA) or Actual Property Agency (REA) examination, or equal; Exclusive brokers are extra keen to share listing information thus making certain the widest doable coverage inside the real estate community via Multiple Listings and Networking. Accepting a severe provide is simpler since your agent is totally conscious of all advertising activity related with your property. This reduces your having to check with a number of agents for some other offers. Price control is easily achieved. Paint work in good restore-discuss with your Property Marketing consultant if main works are still to be done. Softening in residential property prices proceed, led by 2.8 per cent decline within the index for Remainder of Central Region
Once you place down the one per cent choice price to carry down a non-public property, it's important to accept its situation as it is whenever you move in – faulty air-con, choked rest room and all. Get round this by asking your agent to incorporate a ultimate inspection clause within the possibility-to-buy letter. HDB flat patrons routinely take pleasure in this security net. "There's a ultimate inspection of the property two days before the completion of all HDB transactions. If the air-con is defective, you can request the seller to repair it," says Kelvin.
15.6.1 As the agent is an intermediary, generally, as soon as the principal and third party are introduced right into a contractual relationship, the agent drops out of the image, subject to any problems with remuneration or indemnification that he could have against the principal, and extra exceptionally, against the third occasion. Generally, agents are entitled to be indemnified for all liabilities reasonably incurred within the execution of the brokers´ authority.
To achieve the very best outcomes, you must be always updated on market situations, including past transaction information and reliable projections. You could review and examine comparable homes that are currently available in the market, especially these which have been sold or not bought up to now six months. You'll be able to see a pattern of such report by clicking here It's essential to defend yourself in opposition to unscrupulous patrons. They are often very skilled in using highly unethical and manipulative techniques to try and lure you into a lure. That you must also protect your self, your loved ones, and personal belongings as you'll be serving many strangers in your home. Sign a listing itemizing of all of the objects provided by the proprietor, together with their situation. HSR Prime Recruiter 2010
- Many property agents need to declare for the PIC grant in Singapore. However, not all of them know find out how to do the correct process for getting this PIC scheme from the IRAS. There are a number of steps that you need to do before your software can be approved.
Naturally, you will have to pay a safety deposit and that is usually one month rent for annually of the settlement. That is the place your good religion deposit will likely be taken into account and will kind part or all of your security deposit. Anticipate to have a proportionate amount deducted out of your deposit if something is discovered to be damaged if you move out. It's best to you'll want to test the inventory drawn up by the owner, which can detail all objects in the property and their condition. If you happen to fail to notice any harm not already mentioned within the inventory before transferring in, you danger having to pay for it yourself.
In case you are in search of an actual estate or Singapore property agent on-line, you simply should belief your intuition. It's because you do not know which agent is nice and which agent will not be. Carry out research on several brokers by looking out the internet. As soon as if you end up positive that a selected agent is dependable and reliable, you can choose to utilize his partnerise in finding you a home in Singapore. Most of the time, a property agent is taken into account to be good if he or she locations the contact data on his website. This may mean that the agent does not mind you calling them and asking them any questions relating to new properties in singapore in Singapore. After chatting with them you too can see them in their office after taking an appointment.
Have handed an trade examination i.e Widespread Examination for House Brokers (CEHA) or Actual Property Agency (REA) examination, or equal; Exclusive brokers are extra keen to share listing information thus making certain the widest doable coverage inside the real estate community via Multiple Listings and Networking. Accepting a severe provide is simpler since your agent is totally conscious of all advertising activity related with your property. This reduces your having to check with a number of agents for some other offers. Price control is easily achieved. Paint work in good restore-discuss with your Property Marketing consultant if main works are still to be done. Softening in residential property prices proceed, led by 2.8 per cent decline within the index for Remainder of Central Region
Once you place down the one per cent choice price to carry down a non-public property, it's important to accept its situation as it is whenever you move in – faulty air-con, choked rest room and all. Get round this by asking your agent to incorporate a ultimate inspection clause within the possibility-to-buy letter. HDB flat patrons routinely take pleasure in this security net. "There's a ultimate inspection of the property two days before the completion of all HDB transactions. If the air-con is defective, you can request the seller to repair it," says Kelvin.
15.6.1 As the agent is an intermediary, generally, as soon as the principal and third party are introduced right into a contractual relationship, the agent drops out of the image, subject to any problems with remuneration or indemnification that he could have against the principal, and extra exceptionally, against the third occasion. Generally, agents are entitled to be indemnified for all liabilities reasonably incurred within the execution of the brokers´ authority.
To achieve the very best outcomes, you must be always updated on market situations, including past transaction information and reliable projections. You could review and examine comparable homes that are currently available in the market, especially these which have been sold or not bought up to now six months. You'll be able to see a pattern of such report by clicking here It's essential to defend yourself in opposition to unscrupulous patrons. They are often very skilled in using highly unethical and manipulative techniques to try and lure you into a lure. That you must also protect your self, your loved ones, and personal belongings as you'll be serving many strangers in your home. Sign a listing itemizing of all of the objects provided by the proprietor, together with their situation. HSR Prime Recruiter 2010.
External links
- ↑ Andrews, Larry C.; Special functions of mathematics for engineers
- ↑ 2.0 2.1 Greene, William H.; Econometric Analysis (fifth edition), Prentice-Hall, 1993, p. 926, fn. 11
- ↑ 3.0 3.1 3.2 W. J. Cody, "Algorithm 715: SPECFUN—A portable FORTRAN package of special function routines and test drivers," ACM Trans. Math. Soft. 19, pp. 22–32 (1993).
- ↑ M. R. Zaghloul, "On the calculation of the Voigt line profile: a single proper integral with a damped sine integrand," Monthly Notices of the Royal Astronomical Society '375, pp. 1043–1048 (2007).
- ↑ Van Zeghbroeck, Bart; Principles of Semiconductor Devices, University of Colorado, 2011. [1]
- ↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - ↑ Template:Cite web
- ↑ Numerical Recipes in Fortran 77: The Art of Scientific Computing (ISBN 0-521-43064-X), 1992, page 214, Cambridge University Press.
- ↑ http://hackage.haskell.org/package/erf
- ↑ http://commons.apache.org/math
- ↑ 11.0 11.1 11.2 One of the biggest reasons investing in a Singapore new launch is an effective things is as a result of it is doable to be lent massive quantities of money at very low interest rates that you should utilize to purchase it. Then, if property values continue to go up, then you'll get a really high return on funding (ROI). Simply make sure you purchase one of the higher properties, reminiscent of the ones at Fernvale the Riverbank or any Singapore landed property Get Earnings by means of Renting
In its statement, the singapore property listing - website link, government claimed that the majority citizens buying their first residence won't be hurt by the new measures. Some concessions can even be prolonged to chose teams of consumers, similar to married couples with a minimum of one Singaporean partner who are purchasing their second property so long as they intend to promote their first residential property. Lower the LTV limit on housing loans granted by monetary establishments regulated by MAS from 70% to 60% for property purchasers who are individuals with a number of outstanding housing loans on the time of the brand new housing purchase. Singapore Property Measures - 30 August 2010 The most popular seek for the number of bedrooms in Singapore is 4, followed by 2 and three. Lush Acres EC @ Sengkang
Discover out more about real estate funding in the area, together with info on international funding incentives and property possession. Many Singaporeans have been investing in property across the causeway in recent years, attracted by comparatively low prices. However, those who need to exit their investments quickly are likely to face significant challenges when trying to sell their property – and could finally be stuck with a property they can't sell. Career improvement programmes, in-house valuation, auctions and administrative help, venture advertising and marketing, skilled talks and traisning are continuously planned for the sales associates to help them obtain better outcomes for his or her shoppers while at Knight Frank Singapore. No change Present Rules
Extending the tax exemption would help. The exemption, which may be as a lot as $2 million per family, covers individuals who negotiate a principal reduction on their existing mortgage, sell their house short (i.e., for lower than the excellent loans), or take part in a foreclosure course of. An extension of theexemption would seem like a common-sense means to assist stabilize the housing market, but the political turmoil around the fiscal-cliff negotiations means widespread sense could not win out. Home Minority Chief Nancy Pelosi (D-Calif.) believes that the mortgage relief provision will be on the table during the grand-cut price talks, in response to communications director Nadeam Elshami. Buying or promoting of blue mild bulbs is unlawful.
A vendor's stamp duty has been launched on industrial property for the primary time, at rates ranging from 5 per cent to 15 per cent. The Authorities might be trying to reassure the market that they aren't in opposition to foreigners and PRs investing in Singapore's property market. They imposed these measures because of extenuating components available in the market." The sale of new dual-key EC models will even be restricted to multi-generational households only. The models have two separate entrances, permitting grandparents, for example, to dwell separately. The vendor's stamp obligation takes effect right this moment and applies to industrial property and plots which might be offered inside three years of the date of buy. JLL named Best Performing Property Brand for second year running
The data offered is for normal info purposes only and isn't supposed to be personalised investment or monetary advice. Motley Fool Singapore contributor Stanley Lim would not personal shares in any corporations talked about. Singapore private home costs increased by 1.eight% within the fourth quarter of 2012, up from 0.6% within the earlier quarter. Resale prices of government-built HDB residences which are usually bought by Singaporeans, elevated by 2.5%, quarter on quarter, the quickest acquire in five quarters. And industrial property, prices are actually double the levels of three years ago. No withholding tax in the event you sell your property. All your local information regarding vital HDB policies, condominium launches, land growth, commercial property and more
There are various methods to go about discovering the precise property. Some local newspapers (together with the Straits Instances ) have categorised property sections and many local property brokers have websites. Now there are some specifics to consider when buying a 'new launch' rental. Intended use of the unit Every sale begins with 10 p.c low cost for finish of season sale; changes to 20 % discount storewide; follows by additional reduction of fiftyand ends with last discount of 70 % or extra. Typically there is even a warehouse sale or transferring out sale with huge mark-down of costs for stock clearance. Deborah Regulation from Expat Realtor shares her property market update, plus prime rental residences and houses at the moment available to lease Esparina EC @ Sengkang - ↑ Error Function and Fresnel Integrals, SciPy v0.13.0 Reference Guide.
- ↑ Many property agents need to declare for the PIC grant in Singapore. However, not all of them know find out how to do the correct process for getting this PIC scheme from the IRAS. There are a number of steps that you need to do before your software can be approved.
Naturally, you will have to pay a safety deposit and that is usually one month rent for annually of the settlement. That is the place your good religion deposit will likely be taken into account and will kind part or all of your security deposit. Anticipate to have a proportionate amount deducted out of your deposit if something is discovered to be damaged if you move out. It's best to you'll want to test the inventory drawn up by the owner, which can detail all objects in the property and their condition. If you happen to fail to notice any harm not already mentioned within the inventory before transferring in, you danger having to pay for it yourself.
In case you are in search of an actual estate or Singapore property agent on-line, you simply should belief your intuition. It's because you do not know which agent is nice and which agent will not be. Carry out research on several brokers by looking out the internet. As soon as if you end up positive that a selected agent is dependable and reliable, you can choose to utilize his partnerise in finding you a home in Singapore. Most of the time, a property agent is taken into account to be good if he or she locations the contact data on his website. This may mean that the agent does not mind you calling them and asking them any questions relating to new properties in singapore in Singapore. After chatting with them you too can see them in their office after taking an appointment.
Have handed an trade examination i.e Widespread Examination for House Brokers (CEHA) or Actual Property Agency (REA) examination, or equal; Exclusive brokers are extra keen to share listing information thus making certain the widest doable coverage inside the real estate community via Multiple Listings and Networking. Accepting a severe provide is simpler since your agent is totally conscious of all advertising activity related with your property. This reduces your having to check with a number of agents for some other offers. Price control is easily achieved. Paint work in good restore-discuss with your Property Marketing consultant if main works are still to be done. Softening in residential property prices proceed, led by 2.8 per cent decline within the index for Remainder of Central Region
Once you place down the one per cent choice price to carry down a non-public property, it's important to accept its situation as it is whenever you move in – faulty air-con, choked rest room and all. Get round this by asking your agent to incorporate a ultimate inspection clause within the possibility-to-buy letter. HDB flat patrons routinely take pleasure in this security net. "There's a ultimate inspection of the property two days before the completion of all HDB transactions. If the air-con is defective, you can request the seller to repair it," says Kelvin.
15.6.1 As the agent is an intermediary, generally, as soon as the principal and third party are introduced right into a contractual relationship, the agent drops out of the image, subject to any problems with remuneration or indemnification that he could have against the principal, and extra exceptionally, against the third occasion. Generally, agents are entitled to be indemnified for all liabilities reasonably incurred within the execution of the brokers´ authority.
To achieve the very best outcomes, you must be always updated on market situations, including past transaction information and reliable projections. You could review and examine comparable homes that are currently available in the market, especially these which have been sold or not bought up to now six months. You'll be able to see a pattern of such report by clicking here It's essential to defend yourself in opposition to unscrupulous patrons. They are often very skilled in using highly unethical and manipulative techniques to try and lure you into a lure. That you must also protect your self, your loved ones, and personal belongings as you'll be serving many strangers in your home. Sign a listing itemizing of all of the objects provided by the proprietor, together with their situation. HSR Prime Recruiter 2010