Montgomery curve: Difference between revisions
en>Tassedethe m WPCleaner v1.14 - Repaired 1 link to disambiguation page - (You can help) - Peter Montgomery |
en>Bender235 No edit summary |
||
| Line 1: | Line 1: | ||
{{Cleanup|date=February 2010}} | |||
The '''tripling-oriented Doche–Icart–Kohel curve''' is a form of an [[elliptic curve]] that has been used lately in [[cryptography]]; it is a particular type of [[elliptic curve|Weierstrass curve]]. At certain conditions some [[operation (mathematics)|operations]], as adding, doubling or tripling points, are faster to compute using this form. | |||
The Tripling oriented Doche–Icart–Kohel curve, often called with the abbreviation '''3DIK''' has been introduced by Christophe Doche, Thomas Icart, and David R. Kohel in <ref>Christophe Doche, Thomas Icart, and David R. Kohel, ''Efficient Scalar Multiplication by Isogeny Decompositions''</ref> | |||
==Definition== | |||
[[Image:Tripling oriented.svg|300px|right|thumb|A tripling-oriented Doche–Icart–Kohel curve of equation <math>y^2=x^3 - 3x^2 - 6x - 3</math>]] | |||
Let <math>K</math> be a [[field (mathematics)|field]] of [[characteristic (algebra)|characteristic]] different form 2 and 3. | |||
An elliptic curve in '''tripling oriented Doche–Icart–Kohel form''' is defined by the [[equation]]: | |||
: <math>T_a\ :\ y^2 = x^3 + 3a(x+1)^2 </math> | |||
with <math>a\in K</math>. | |||
A general [[point (geometry)|point]] ''P'' on <math>T_{a}</math> has [[affine space|affine coordinates]] <math>(x,y)</math>. The "point at infinity" represents the [[identity element|neutral element]] for the group law and it is written in [[projective space|projective coordinates]] as O = (0:1:0). The negation of a point ''P'' = (''x'', ''y'') with respect to this neutral element is −''P'' = (''x'', −''y''). | |||
==The group law== | |||
Consider an elliptic curve in the Tripling-oriented Doche-Icart-Kohel form in [[affine space|affine coordinates]]: | |||
: <math>T_a\ :\ y^2= x^3 + 3a(x+1)^2</math> | |||
with <math>a\neq 0, \frac{9}{4}</math>. | |||
As in other forms of elliptic curves, it is possible to define some "operations" between points, such as adding points, or doubling (See also [[elliptic curve#The group law|The group law]]). | |||
In the following sections formulas to add, negate and doubling points are given. | |||
The addition and doubling formulas are often used for other operations: given a point ''P'' on an elliptic curve it is possible to compute ''[n]P'', where ''n'' is an [[integer]], using addition and doubling; computing multiples of points is important in [[elliptic curve cryptography]] and in [[Lenstra elliptic curve factorization]]. | |||
===Addition=== | |||
Given <math>P_1=(x_1,y_1)</math> and <math>P_2=(x_2,y_2)</math> on <math>T_{a}</math>, the point <math>P_3=(x_3,y_3)=P_1+P_2</math> has coordinates: | |||
: <math> | |||
x_3 = \frac{(-{x_1}^3+(x_2-3a){x_1}^2+({x_2}^2+6ax_2)x_1+({y_1}^2-2{y_2}{y_1}+(-{x_2}^3-3a{x_2}^2+{y_2}^2)))}{({x_1}^2-2{x_2}{x_1}+{x_2}^2)} | |||
</math> | |||
: <math> | |||
y_3 = \frac{((-y_1+2y_2){x_1}^3+(-3ay_1+(-3y_2x_2+3ay_2)){x_1}^2+((3{x_2}^2+6ax_2)y_1-6ay_2x_2)x_1+({y_1}^3-3y_2{y_1}^2+(-2{x_2}^3-3a{x_2}^2+3{y_2}^2)y_1+(y_2{x_2}^3+3ay_2{x_2}^2-{y_2}^3)))}{(-{x_1}^3+3{x_2}{x_1}^2-3{x_2}^2x_1+{x_2}^3)} | |||
</math> | |||
===Doubling=== | |||
Given a point <math>P_1=(x_1,y_1)</math> on <math>T_{a}</math>, the point <math>P_3=(x_3,y_3)=2P_1</math> has coordinates: | |||
: <math> | |||
x_3 = \frac{9}{4{y_1}^2{x_1}^4}+\frac{9}{{y_1}^2a{x_1}^3}+(\frac{9}{{y_1}^2a^2}+\frac{9}{{y_1}^2a}){x_1}^2+(\frac{18}{{y_1}^2a^2}-2)x_1+\frac{9}{{y_1}^2a^2-3a} | |||
</math> | |||
: <math> | |||
y_3 = -\frac{27}{8{y_1}^3{x_1}^6}-\frac{81}{4{y_1}^3a{x_1}^5}+(-\frac{81}{2{y_1}^3a^2}-\frac{81}{4{y_1}^3a}){x_1}^4+(-\frac{27}{{y_1}^3a^3}-\frac{81}{{y_1}^3a^2}+\frac{9}{2y_1}){x_1}^3+(-\frac{81}{{y_1}^3a^3}-\frac{81}{2{y_1}^3}a^2+\frac{27}{2y_1a}){x_1}2+(-\frac{81}{{y_1}^3a^3}+\frac{9}{y_1a^2}+\frac{9}{y_1a})x_1+(-\frac{27}{{y_1}^3a^3}+\frac{9}{y_1a^2}-y_1) | |||
</math> | |||
===Negation=== | |||
Given a point <math>P_1=(x_1,y_1)</math> on <math>T_{a}</math>, its [[additive inverse|negation]] with respect to the neutral element <math>(0:1:0)</math> is <math>-P_1=(x_1,-y_1)</math>. | |||
There are also other formulas given in <ref>Christophe Doche, Thomas Icart, and David R. Kohel, ''Efficient Scalar Multiplication by Isogeny Decompositions'', pag 198-199</ref> for Tripling-oriented Doche–Icart–Kohel curves for fast tripling operation and mixed-addition. | |||
==New Jacobian coordinates== | |||
For computing on these curves points are usually represented in '''new Jacobian coordinates''' ('''J<sup>n</sup>'''): | |||
a point in the new Jacobian coordinates is of the form <math>P=(X : Y : Z : Z^2)</math>; moreover: | |||
: <math>P= (X:Y:Z:Z^{2}) = (\lambda^{2}X:\lambda^{3}Y:\lambda Z:\lambda^{2}Z^{2}),</math> | |||
for any <math>\lambda \in K</math>. | |||
This means, for example, that the point <math>P=(X:Y:Z:Z^2)</math> and the point <math>Q=(4X:8Y:2Z:4Z^2)</math> (for <math>\lambda=2</math>) are actually the same. | |||
So, an [[affine space|affine point]] <math>P=(x,y)</math> on <math>T_{a}</math> is written in the new Jacobian coordinates as <math>P=(X : Y : Z : Z^2)</math>, where <math>x = X/Z^2</math> and <math>y = Y/Z^3</math>; in this way, the equation for <math>T_{a}</math> becomes: | |||
: <math>T_a\ :\ Y^2 = X^3 + 3aZ^2(X+Z^2)^2.</math> | |||
The term <math>Z^2</math> of a point on the curve makes the mixed [[Elliptic curve#The group law|addition]] (that is the addition between two points in different [[coordinate system|systems of coordinates]]) more efficient. | |||
The [[identity element|neutral element]] in new Jacobian coordinates is <math>(1:1:0:0)</math>. | |||
==Algorithms and examples== | |||
===Addition=== | |||
The following algorithm represents the sum of two points <math>P_1</math> and <math>P_2</math> on an elliptic curve in the Tripling-oriented Doche-Icart-Kohel form. The result is a point <math>P_3=(X_3,Y_3,Z_3,ZZ_3)</math>. | |||
It is assumed that <math>Z_2=1</math> and that <math>a_3=3a</math>. | |||
The cost of this implementation is 7M + 4S + 1*a3 + 10add + 3*2 + 1*4, where M indicates the multiplications, S the squarings, a3 indicates the multiplication by the constant a<sub>3</sub>, add represents the number of additions required. | |||
<math>A = X_2ZZ_1</math> | |||
<math>B = Y_2ZZ_1Z_1</math> | |||
<math>C = X_1-A</math> | |||
<math>D = 2(Y_1-B)</math> | |||
<math>F = C^2</math> | |||
<math>F_4 = 4F</math> | |||
<math>Z_3 = (Z_1+C)^2-ZZ_1-F</math> | |||
<math>E = {Z_3}^2</math> | |||
<math>G = CF_4</math> | |||
<math>H = AF_4</math> | |||
<math>X_3 = D^2-G-2H-a_3E</math> | |||
<math>Y_3 = D(H-X_3)-2BG</math> | |||
<math>ZZ_3 = E</math> | |||
====Example==== | |||
Let <math>P_1=(1,\sqrt{13})</math> and <math>P_2=(0,\sqrt{3})</math> affine points on the elliptic curve over <math>\mathbb{R}</math>: | |||
<math>y^2 = x^3 +3(x+1)^2</math>. | |||
Then: | |||
<math>A = X_2ZZ_1 = 0</math> | |||
<math>B = Y_2ZZ_1Z_1 = \sqrt{3}</math> | |||
<math>C = X_1-A = 1</math> | |||
<math>D = 2(Y_1-B) = 2(\sqrt{13}-\sqrt{3})</math> | |||
<math>F = C^2 = 1</math> | |||
<math>F_4 = 4F = 4</math> | |||
<math>Z_3 = (Z_1+C)^2-ZZ_1-F = 2</math> | |||
<math>E = {Z_3}^2 = 4</math> | |||
<math>G = CF_4 = 4</math> | |||
<math>H = AF_4 = 0</math> | |||
<math>X_3 = D^2-G-2H-a_3E = 48-8\sqrt{39}</math> | |||
<math>Y_3 = D(H-X_3)-2BG = 296\sqrt{3}-144\sqrt{13}</math> | |||
<math>ZZ_3 = E = 4</math> | |||
Notice that in this case <math>Z_1=Z_2=1</math>. | |||
The resulting point is <math>P_3 = (X_3,Y_3,Z_3,ZZ_3) = (48-8\sqrt{39},296\sqrt{3}-144\sqrt{13},2,4)</math>, that in affine coordinates is <math>P_3=(12-2\sqrt{39},37\sqrt{3}-18\sqrt{13})</math>. | |||
===Doubling=== | |||
The following algorithm represents the doubling of a point <math>P_1</math> on an elliptic curve in the Tripling-oriented Doche-Icart-Kohel form. | |||
It is assumed that <math>a_3=3a</math>, <math>a_2=2a</math>. | |||
The cost of this implementation is 2M + 7S + 1*a2 + 1*a3 + 12add + 2*2 + 1*3 + 1*8; here M represents the multiplications, S the squarings, a2 and a3 indicates the multiplications by the constants a<sub>2</sub> and a<sub>3</sub> respectively, and add indicates the additions. | |||
<math>A = {X_1}^2</math> | |||
<math>B = a_2ZZ_1(X_1+ZZ_1)</math> | |||
<math>C = 3(A+B)</math> | |||
<math>D = {Y_1}^2</math> | |||
<math>E = D^2</math> | |||
<math>Z_3 = (Y_1+Z_1)^2-D-ZZ_1</math> | |||
<math>ZZ_3 = Z_3^2</math> | |||
<math>F = 2((X_1+D)^2-A-E)</math> | |||
<math>X_3 = C^2-a_3ZZ_3-2F</math> | |||
<math>Y_3 = C(F-X_3)-8E</math> | |||
====Example==== | |||
Let <math>P_1=(0,\sqrt{3})</math> be a point on <math>y^2 = x^3 + 3(x+1)^2</math>. | |||
Then: | |||
<math>A = {X_1}^2 = 0</math> | |||
<math>B = a_2ZZ_1(X_1+ZZ_1) = 2</math> | |||
<math>C = 3(A+B) = 6</math> | |||
<math>D = {Y_1}^2 = 3</math> | |||
<math>E = D^2 = 9</math> | |||
<math>Z_3 = (Y_1+Z_1)^2-D-ZZ_1 = 2\sqrt{3}</math> | |||
<math>ZZ_3 = Z_3^2 = 12</math> | |||
<math>F = 2((X_1+D)^2-A-E) = 0</math> | |||
<math>X_3 = C^2-a_3ZZ_3-2F = 0</math> | |||
<math>Y_3 = C(F-X_3)-8E = -72</math> | |||
Notice that here the point is in affine coordinates, so <math>Z_1=1</math>. | |||
The resulting point is <math>P_3=(0,-72,2\sqrt{3},12)</math>, that in affine coordinates is <math>P_3 = (0,-\sqrt{3})</math>. | |||
==Equivalence with Weierstrass form== | |||
Any elliptic curve is [[birational geometry|birationally equivalent]] to another written in the Weierstrass form. | |||
The following '''[[twisted curves|twisted]] tripling-oriented Doche-Icart-Kohel curve''': | |||
<math>T_{a,\lambda}</math>: <math>y^2 = x^3 + 3\lambda a(x+\lambda)^2</math> | |||
can be transformed into the Weierstrass form by the [[map (mathematics)|map]]: | |||
<math> | |||
(x,y)\mapsto (x-\lambda a, y) | |||
</math>. | |||
In this way <math>T_{a,\lambda}</math> becomes: | |||
<math>y^2 = x^3 - 3{\lambda}^2a(a-2)x + {\lambda}^3a(2a^2-6a+3)</math>. | |||
Conversely, given an elliptic curve in the Weierstrass form: | |||
<math>E_{c,d}</math>: <math>y^2 = x^3 +cx^2 +d</math>, | |||
it is possible to find the "corresponding" Tripling-oriented Doche–Icart–Kohel curve, using the following relation: | |||
<math> | |||
\lambda = \frac{-3d(a-2)}{a(2a^2-6a+3)} | |||
</math> | |||
where <math>a</math> is a [[equation solving|root]] of the polynomial <math>6912a(a-2)^3-j(4a-9)</math>. | |||
Here <math>j=\frac{6912c^3}{4c^3+27d^2}</math> is the [[j-invariant]] of the elliptic curve <math>E_{c,d}</math>. | |||
Notice that in this case the map given is not only a birational equivalence, but an [[isomorphism]] between curves. | |||
==Internal link== | |||
For more informations about the running-time required in a specific case, see [[Table of costs of operations in elliptic curves]] | |||
==External links== | |||
* http://hyperelliptic.org/EFD/g1p/index.html | |||
==Notes== | |||
{{reflist}} | |||
==References== | |||
* {{cite book | |||
| author = Christophe Doche, Thomas Icart, and David R. Kohel | |||
| year = 2006 | |||
| series = appeared at [[PKC (conference)|PKC]] 2006, part of LNCS (Lecture Series in Computer Science) volume number 3958 | |||
| title = Efficient Scalar Multiplication by Isogeny Decompositions | |||
| publisher = Springer Verlag | |||
| pages = 285–352 | |||
| url = http://web.science.mq.edu.au/~doche/isogeny.pdf | |||
}} | |||
* {{cite book | |||
| author = [[Daniel J. Bernstein]], Tanja Lange | |||
| year = 2007 | |||
| series = appeared in G.L. Mullen, D. Panario, I.E. Shparlinski (Eds.), Finite Fields and Applications (Proceedings 8th International Conference, Fq8, Melbourne, Australia, July 9–13, 2007) | |||
| title = Analysis and optimization of elliptic-curve single-scalar multiplication | |||
| publisher = Mathematics Subject Classification | |||
| url = http://www.hyperelliptic.org/EFD/precomp.pdf | |||
}} | |||
* {{cite book | |||
| author = [[Daniel J. Bernstein|D.J.Bernstein]], P.Birkner, T.Lange, and C.Peters | |||
| year = 2007 | |||
| series = apperared in K. Srinathan, C. Pandu Rangan, M. Yung (Eds.), Proceedings of the 8th International Conference on Cryptology in India: Progress in Cryptology (INDOCRYPT 2007) 9–13 December 2007, Chennai, India | |||
| title = Optimizing Double-Base Elliptic-Curve Single-Scalar Multiplication | |||
| publisher = Springer | |||
| url = http://cr.yp.to/antiforgery/doublebase-20071028.pdf | |||
}} | |||
*http://hyperelliptic.org/EFD/g1p/auto-3dik-standard.html | |||
{{DEFAULTSORT:Tripling-oriented Doche-Icart-Kohel curve}} | |||
[[Category:Elliptic curves]] | |||
[[Category:Elliptic curve cryptography]] | |||
Latest revision as of 11:12, 29 December 2013
The tripling-oriented Doche–Icart–Kohel curve is a form of an elliptic curve that has been used lately in cryptography; it is a particular type of Weierstrass curve. At certain conditions some operations, as adding, doubling or tripling points, are faster to compute using this form. The Tripling oriented Doche–Icart–Kohel curve, often called with the abbreviation 3DIK has been introduced by Christophe Doche, Thomas Icart, and David R. Kohel in [1]
Definition
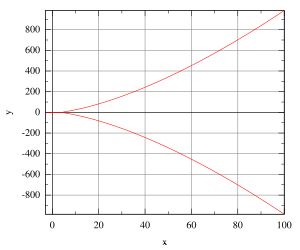
Let be a field of characteristic different form 2 and 3.
An elliptic curve in tripling oriented Doche–Icart–Kohel form is defined by the equation:
A general point P on has affine coordinates . The "point at infinity" represents the neutral element for the group law and it is written in projective coordinates as O = (0:1:0). The negation of a point P = (x, y) with respect to this neutral element is −P = (x, −y).
The group law
Consider an elliptic curve in the Tripling-oriented Doche-Icart-Kohel form in affine coordinates:
As in other forms of elliptic curves, it is possible to define some "operations" between points, such as adding points, or doubling (See also The group law). In the following sections formulas to add, negate and doubling points are given. The addition and doubling formulas are often used for other operations: given a point P on an elliptic curve it is possible to compute [n]P, where n is an integer, using addition and doubling; computing multiples of points is important in elliptic curve cryptography and in Lenstra elliptic curve factorization.
Addition
Given and on , the point has coordinates:
Doubling
Given a point on , the point has coordinates:
Negation
Given a point on , its negation with respect to the neutral element is .
There are also other formulas given in [2] for Tripling-oriented Doche–Icart–Kohel curves for fast tripling operation and mixed-addition.
New Jacobian coordinates
For computing on these curves points are usually represented in new Jacobian coordinates (Jn):
a point in the new Jacobian coordinates is of the form ; moreover:
This means, for example, that the point and the point (for ) are actually the same.
So, an affine point on is written in the new Jacobian coordinates as , where and ; in this way, the equation for becomes:
The term of a point on the curve makes the mixed addition (that is the addition between two points in different systems of coordinates) more efficient.
The neutral element in new Jacobian coordinates is .
Algorithms and examples
Addition
The following algorithm represents the sum of two points and on an elliptic curve in the Tripling-oriented Doche-Icart-Kohel form. The result is a point . It is assumed that and that . The cost of this implementation is 7M + 4S + 1*a3 + 10add + 3*2 + 1*4, where M indicates the multiplications, S the squarings, a3 indicates the multiplication by the constant a3, add represents the number of additions required.
Example
Let and affine points on the elliptic curve over :
Then:
Notice that in this case . The resulting point is , that in affine coordinates is .
Doubling
The following algorithm represents the doubling of a point on an elliptic curve in the Tripling-oriented Doche-Icart-Kohel form. It is assumed that , . The cost of this implementation is 2M + 7S + 1*a2 + 1*a3 + 12add + 2*2 + 1*3 + 1*8; here M represents the multiplications, S the squarings, a2 and a3 indicates the multiplications by the constants a2 and a3 respectively, and add indicates the additions.
Example
Then:
Notice that here the point is in affine coordinates, so . The resulting point is , that in affine coordinates is .
Equivalence with Weierstrass form
Any elliptic curve is birationally equivalent to another written in the Weierstrass form.
The following twisted tripling-oriented Doche-Icart-Kohel curve:
can be transformed into the Weierstrass form by the map:
Conversely, given an elliptic curve in the Weierstrass form:
it is possible to find the "corresponding" Tripling-oriented Doche–Icart–Kohel curve, using the following relation:
where is a root of the polynomial .
Here is the j-invariant of the elliptic curve .
Notice that in this case the map given is not only a birational equivalence, but an isomorphism between curves.
Internal link
For more informations about the running-time required in a specific case, see Table of costs of operations in elliptic curves
External links
Notes
43 year old Petroleum Engineer Harry from Deep River, usually spends time with hobbies and interests like renting movies, property developers in singapore new condominium and vehicle racing. Constantly enjoys going to destinations like Camino Real de Tierra Adentro.
References
- 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - http://hyperelliptic.org/EFD/g1p/auto-3dik-standard.html